25
高校数学解説
歴史上の数学者さん達とコラボしてみた(*´∀`*)
476
0
ガリレオシーケンス
・ガリレオ featuring みゆ
 ガリレオ
ガリレオ
 みゆれお
みゆれお
二平方恒等式
・ディオファントス featuring みゆ
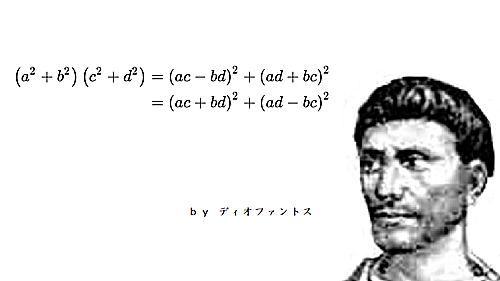 ディオファントス
ディオファントス
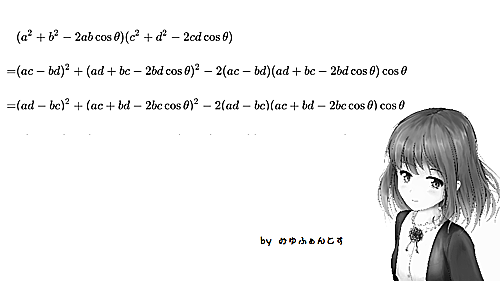 みゆふぁんとす
みゆふぁんとす
円周率を求める公式
・マチン featuring みゆ
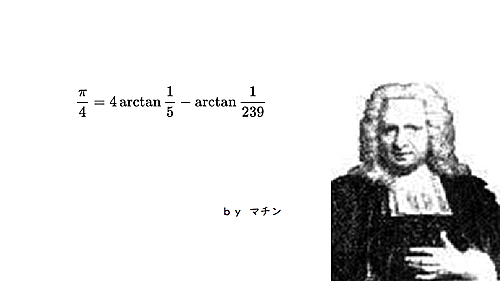 マチン
マチン
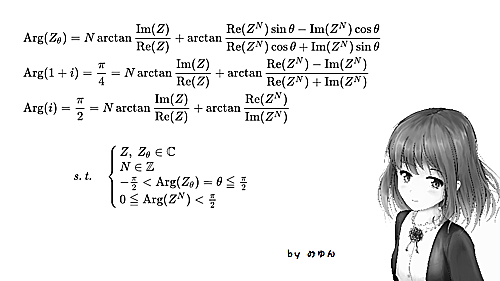 みゆん
みゆん
極座標と直交(斜交)座標の関係式
・オイラー featuring みゆ
 オイラー
オイラー
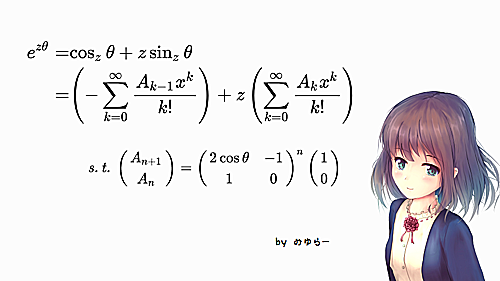 みゆらー
みゆらー
立方和の恒等式
・ラマヌジャン featuring みゆ
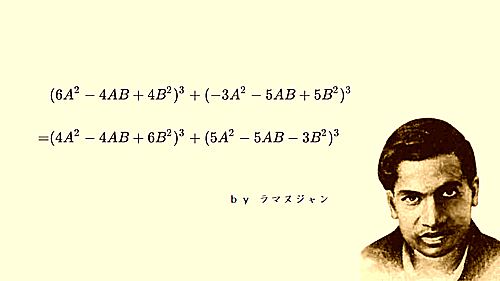 ラマヌジャン
ラマヌジャン
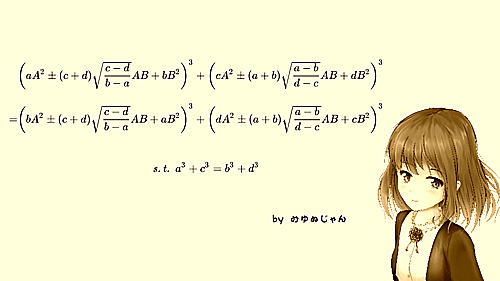 みゆぬじゃん
みゆぬじゃん
投稿日:2020年11月11日
この記事を高評価した人
高評価したユーザはいません
投稿者
みゆ🌹 ฅ^•ω•^ฅ @ 数学を愛する会
586
67437
https://mathlog.info/articles/323
数学を愛する会 副会長 CCO / ガラパゴ数学 開拓者 / 猫舌・甘党・薄味派
コメント
他の人のコメント
コメントはありません。
読み込み中...
読み込み中
