0
高校数学解説
初等的な単振り子のθについての微分方程式導出
173
0
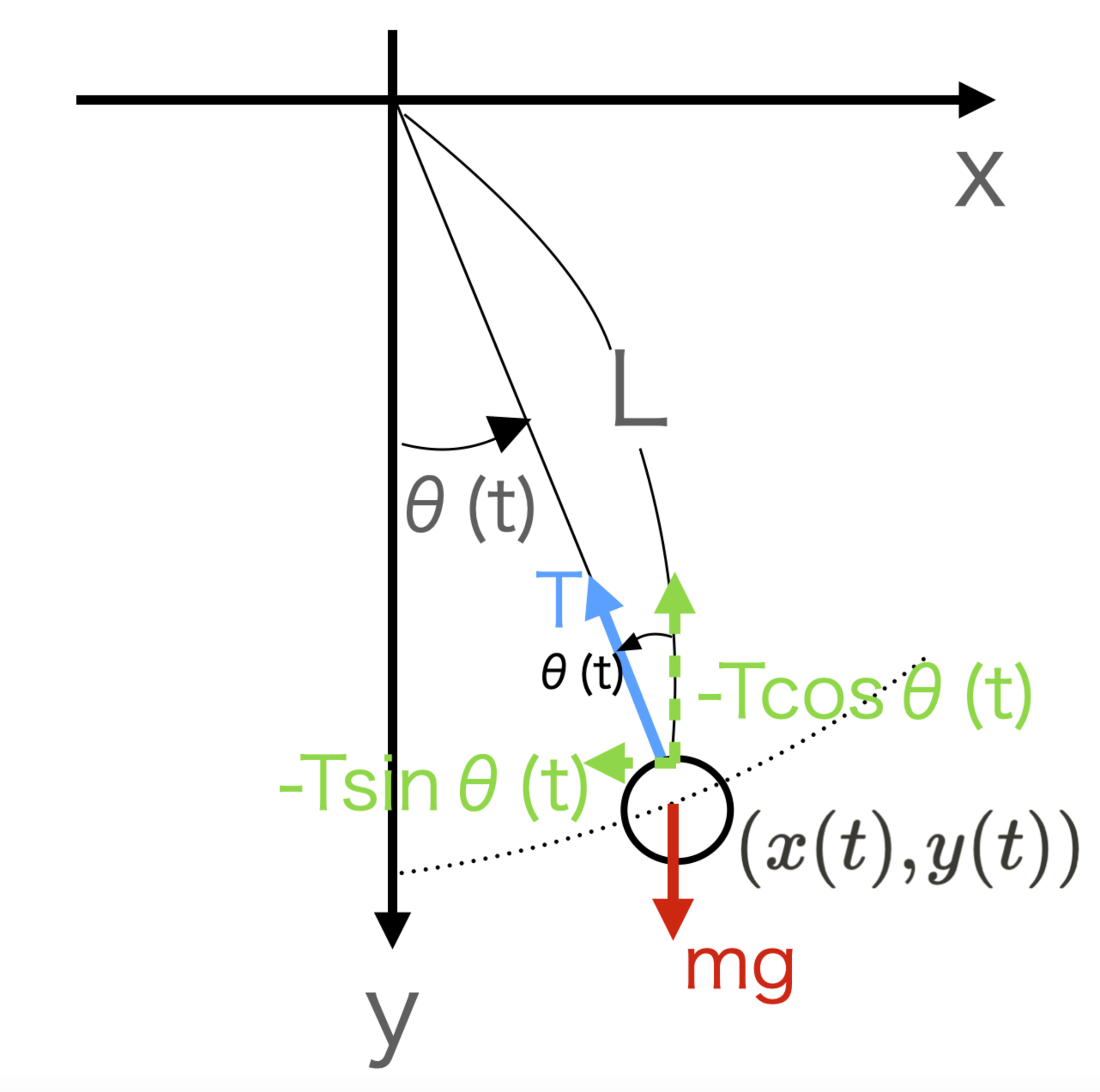 単振り子
単振り子
単振り子の周期(微小振動での近似計算)
ニュートンの運動方程式より
単振り子の周期Tは
proof:
図より、
各々について合成関数の微分をすると、
さらに各々を微分すると、
又、
[(1)式]
ここで、
これは単振動型の微分方程式であるので、
Q.E.D
投稿日:2023年6月23日
この記事を高評価した人
この記事を高評価した人
高評価したユーザはいません
投稿者
投稿者
spaceofstarPhy
0
316
コメント
コメント
他の人のコメント
コメントはありません。
読み込み中...
読み込み中