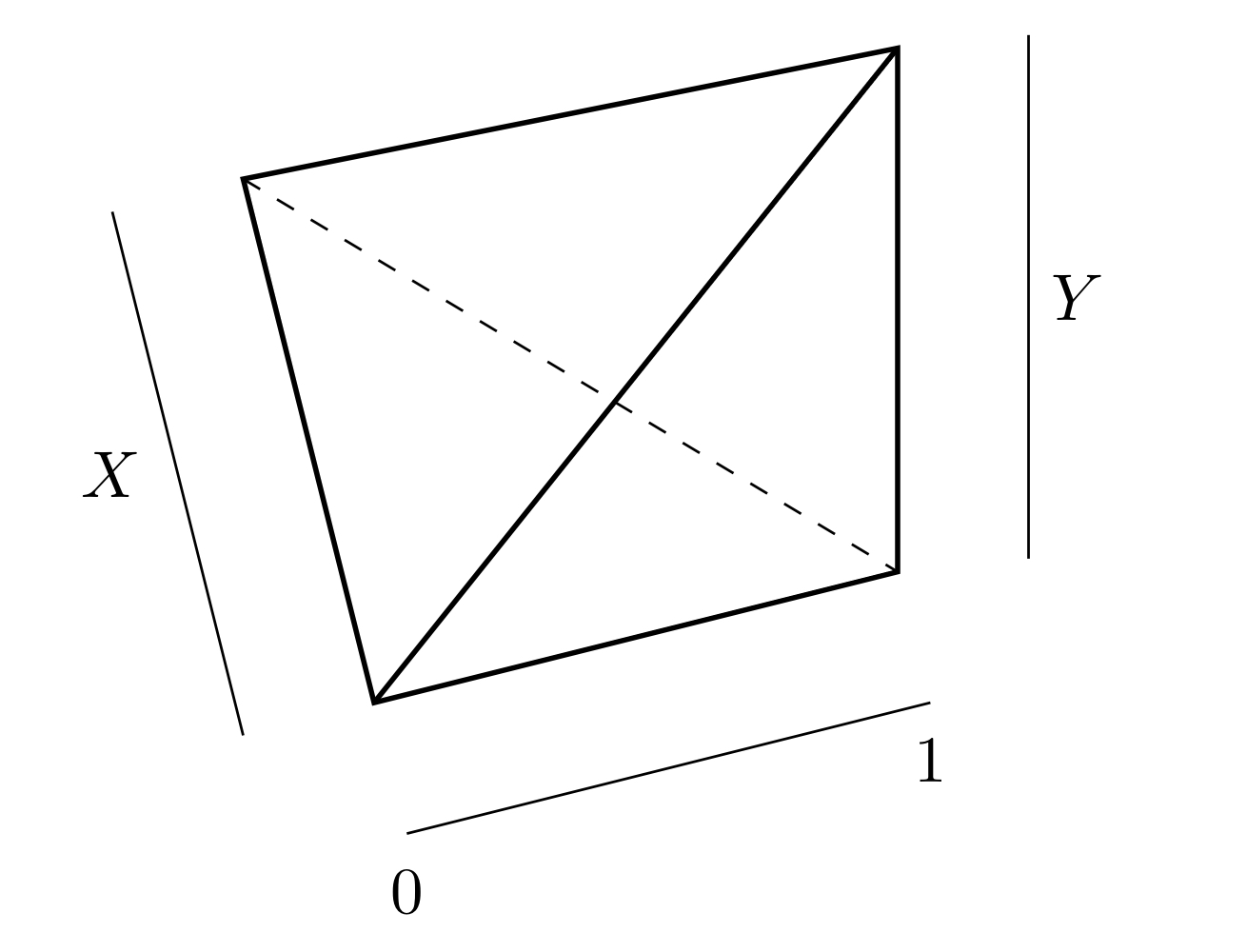
商空間の例
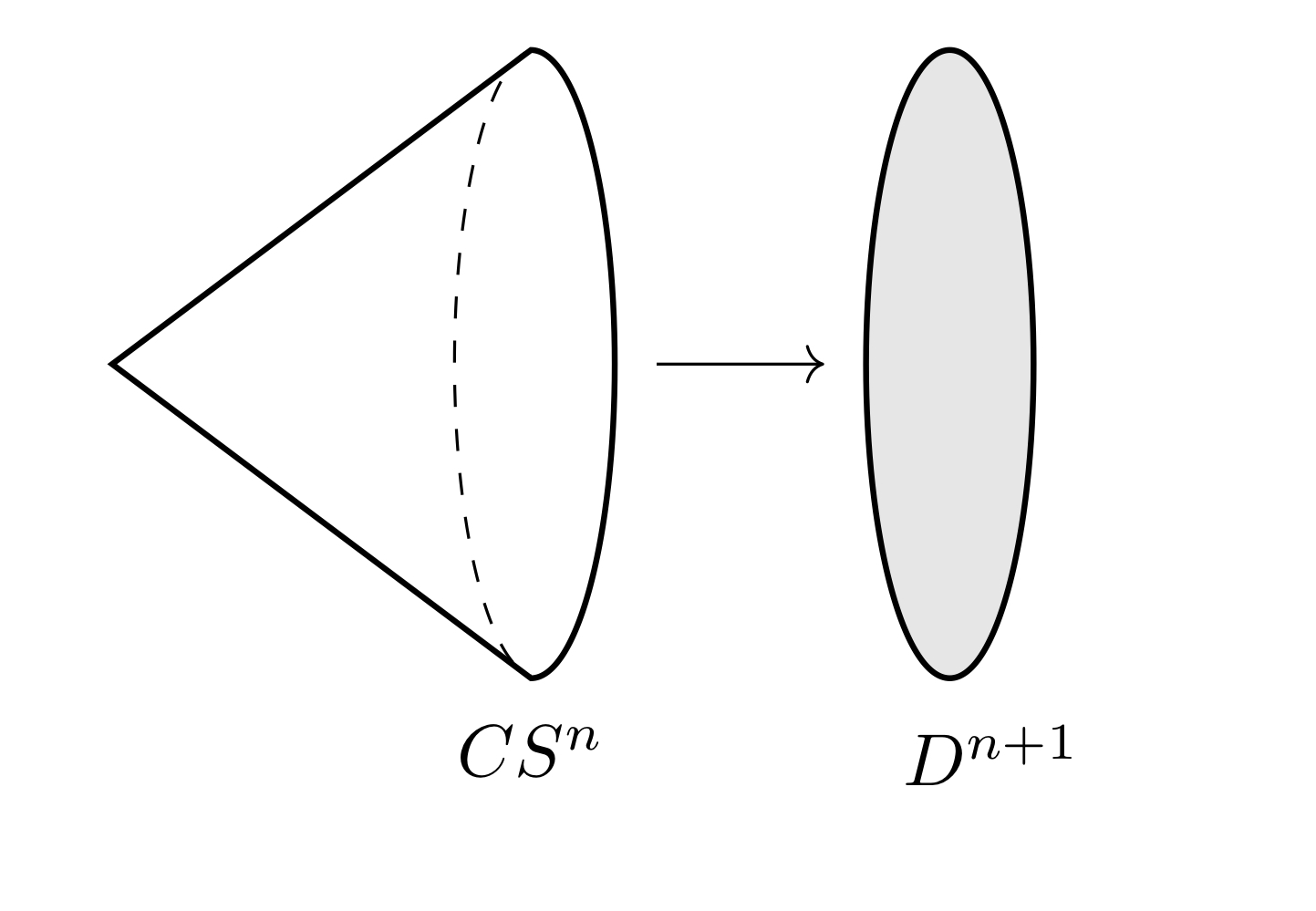
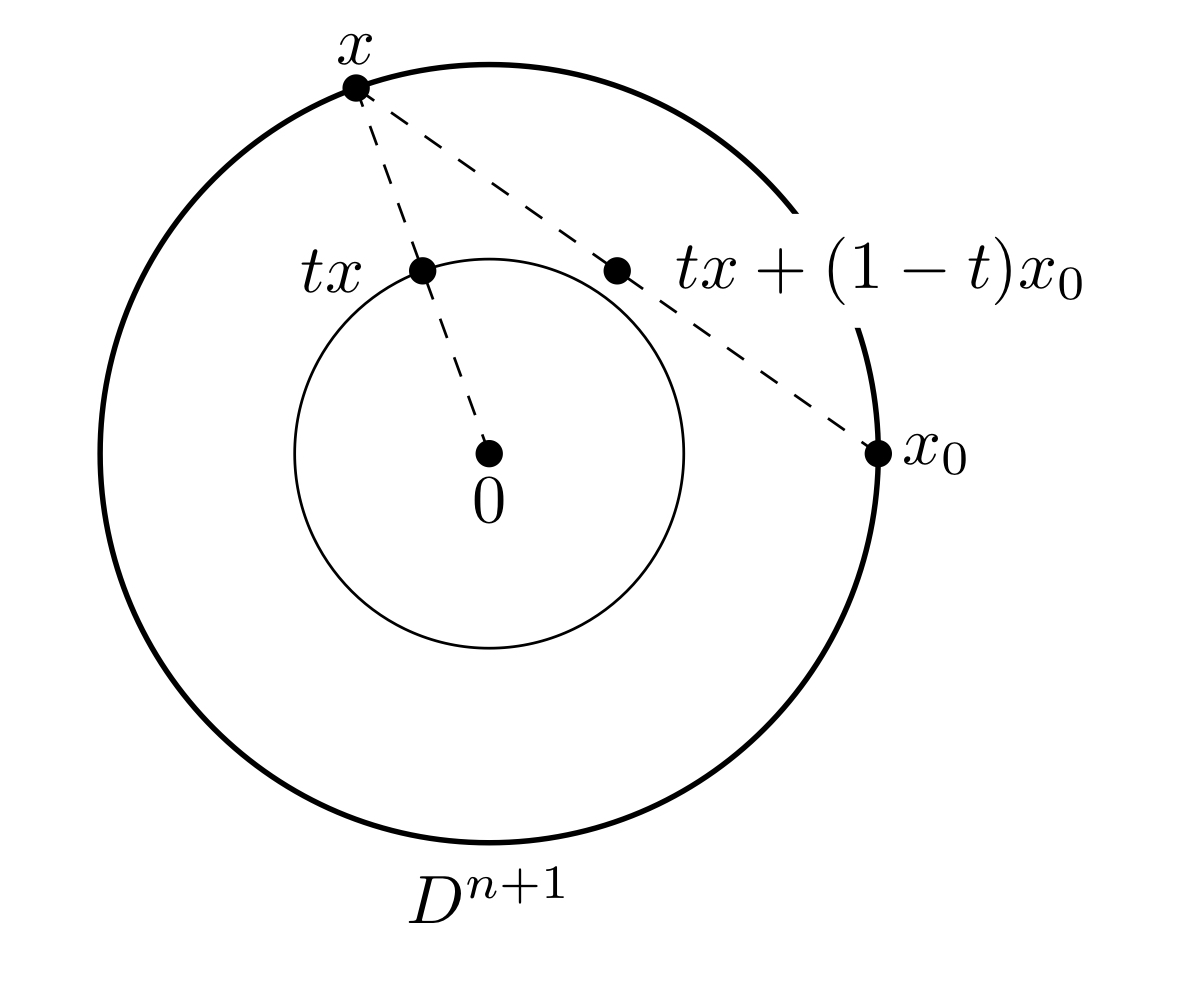
錐
によって生成される同値関係
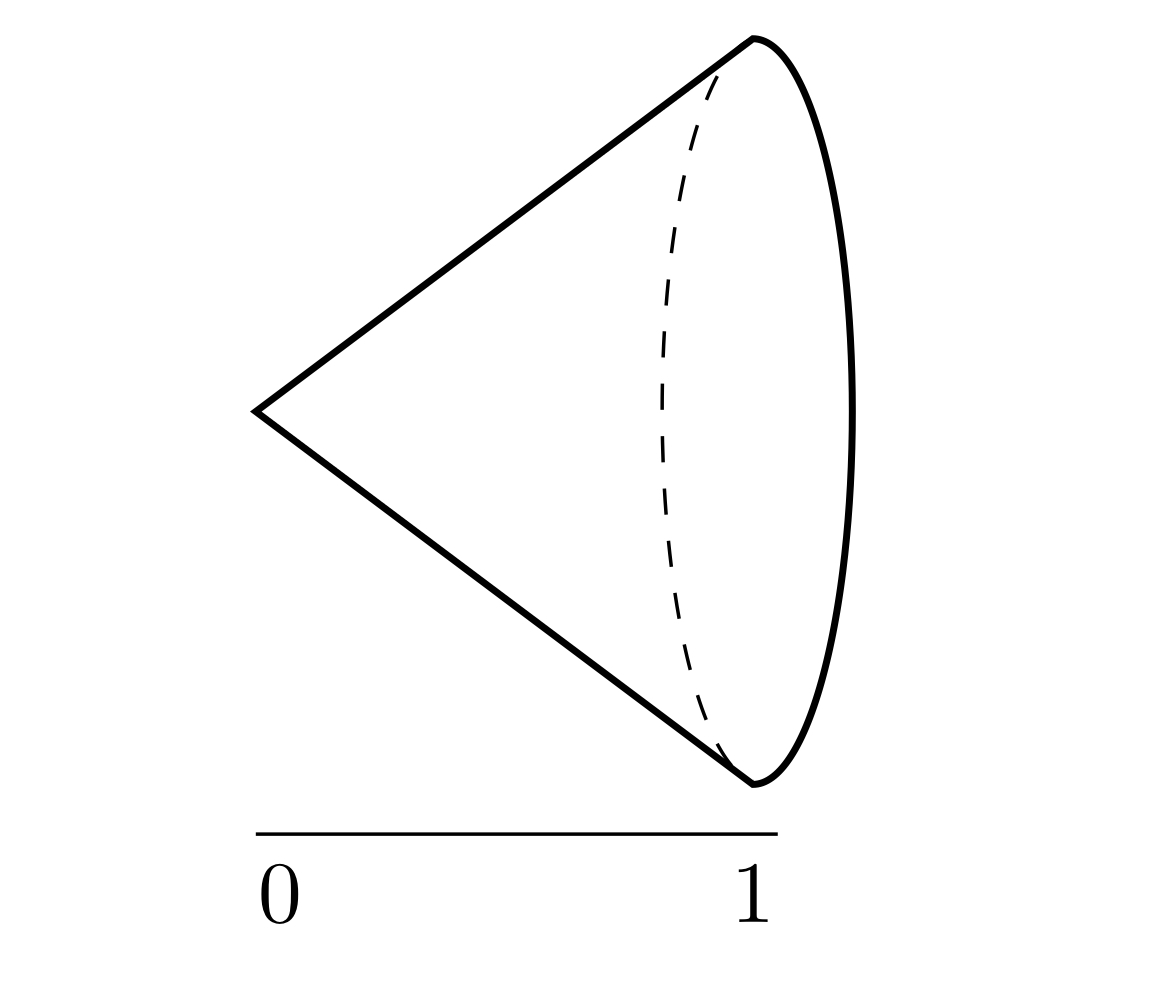 錐
錐
と同相である.以下,この同相により
が成り立つ.
がコンパクト空間
非空閉集合
と同相である.
が成り立つ.また,
によって生成される同値関係に一致することに注意すると以下がわかる:
- 包含写像
- これらは互いの逆写像である.
命題5.3より次を得る:
連続写像
を誘導する.
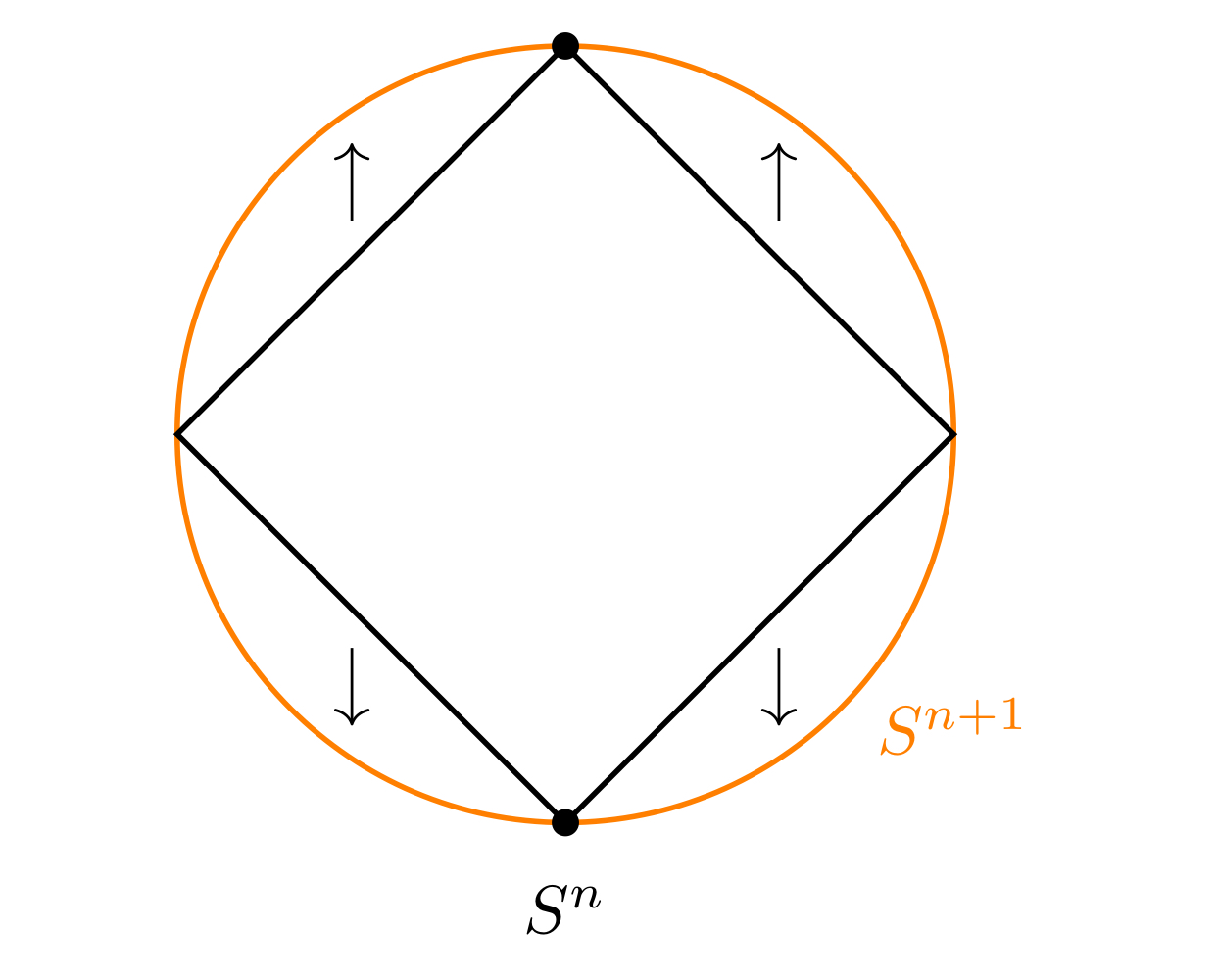
懸垂
によって生成される同値関係
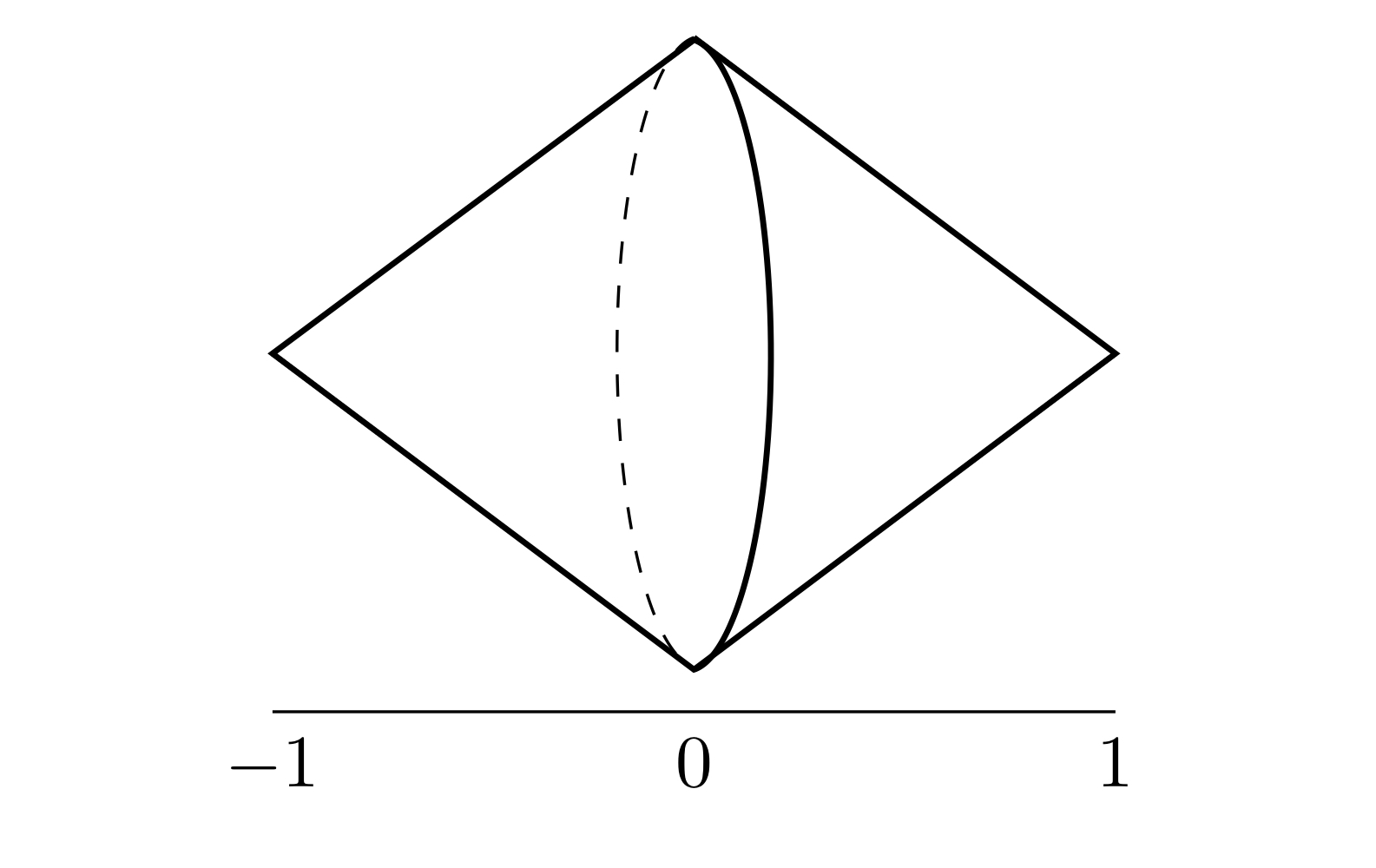 懸垂
懸垂
位相空間
- 同相
- 同相写像
が同相写像
によって生成される同値関係に一致するので,命題5.4より同相
が成り立つ.- (ii)と同様.ただし
となる. - 命題1と前段より明らか.
- 前段と命題5.15よりしたがう.
がコンパクト空間
が成り立つ.
非空閉集合
と同相である.
によって生成される同値関係に一致するので,命題5.4より同相
が成り立つ.
連続写像
を誘導する.
結
によって生成される同値関係
 結
結
と同相である;
と同相である;
と同相である;
が成り立つ.ただし- (i)と同様.
が成り立つ.- 同相写像
が同相写像
非空閉集合
と同相である.
より
によって生成される同値関係に一致するので,命題5.4より同相
が成り立つ.
- 同相写像
が同相 - 連続写像
は連続写像
が
結の結合性について
等化結と誘導結
で生成される同値関係による商空間を
同相
等化位相の普遍性より,各
は連続である.したがって
は連続である.
- 「等化結」および「誘導結」は(おそらく)本稿のみの用語である.
- 位相の定め方より恒等写像
は連続である.
誘導結の結合性
位相空間
連続写像
写像
- 各
が成り立つ.
より,- 任意の
- よって
- また,
が成り立つので,
- 同様にして
よって
連続写像
写像
ただし,
とおいた.このとき,
より,
および
が成り立つ.したがって- 同様にして
よって
および
が成り立つ.
位相空間
が成り立つ.
等化結の結合性:コンパクトハウスドルフ空間の場合
のうち少なくとも一つが成り立つことがわかる.したがって,写像
の連続性および
は同相写像である.
- 命題10,補題11より,
はハウスドルフ空間である.
命題12の証明より次がわかる:
コンパクトハウスドルフ空間
が成り立つ.
命題10(の系)と命題12より
を得る.
コンパクトハウスドルフ空間
が成り立つ.実際,
を得る.
非負整数
が成り立つ.
が成り立つ.
が成り立つ.
等化結の結合性:局所コンパクトハウスドルフ空間の場合(未)
あやしくなってきた.
基点つき空間
- 位相空間
以下,基点つき空間
被約錐・被約懸垂
- 被約錐
- 被約懸垂
- 同相
が成り立つ.
が成り立つ.
が成り立つ.- 前段より
を得る.
が貼り合わさって定まる連続写像- 前段より
が成り立つ. - 前段と命題5.15よりしたがう.
はコンパクト空間
![!FORMULA[325][1004258240][0]をつぶす](https://firebasestorage.googleapis.com/v0/b/mathlog-361213.appspot.com/o/uploads%2Fmathdown%2FWPuxWauRYjZI1frzBiQ0.jpeg?alt=media)
で定める.
で“左右に膨らませる”写像である.したがって,
![!FORMULA[344][-1977159728][0]をつぶす](https://firebasestorage.googleapis.com/v0/b/mathlog-361213.appspot.com/o/uploads%2Fmathdown%2F8Q0xM5LWF64rxxjcpy18.jpeg?alt=media)
命題13,例6,例1より
を得る.
基点つき空間
が成り立つ.
単位閉区間
は全射等化写像である(定理4.11の系).したがって,合成
は全射等化写像である(定理3.7の系).また,同値関係
を一点につぶす同値関係である.同様に
は全射等化写像であり,
が成り立つ.よって,同相写像
が同相
を誘導する.
楔和
によって生成される同値関係による商空間,すなわち部分集合
余積空間の結合性(resp. 可換性)より,楔和の結合性(resp. 可換性)がしたがう.
が成り立つものがただ一つ存在する.
余積空間の普遍性より連続写像
いま
より,
が成り立つ.よって,商空間の普遍性より連続写像
この
より,
が成り立つ.
より,
が成り立つ.
とおくと,これは
商写像を
- 各
とおくと,これは
で定めると,これらは
を定める. - 各
で定めると,
が定まる.
こうして定まる
縮積
を一点につぶして得られる空間を
- とくに
となる. - 積空間の可換性より,縮積の可換性がしたがう.
- 連続写像
は連続写像
が成り立つ.- 連続写像
が成り立つことがわかるので,同相
が成り立つ.
基点つき空間のあいだの連続写像の族
を誘導する.
各
が成り立つので,命題5.3より結論を得る.
- 補題19より,連続写像
が成り立つ.
ところで,同一視
が成り立つことがわかるので,結論を得る.
が成り立つ.
任意の
が成り立つので,全射連続写像
を誘導する:
いま,仮定より
命題21の仮定が成り立つための十分条件として,たとえば次のものがある:
のいづれかが成り立つならば,同相
が成り立つ.
全射連続写像
を考える.
- (1)と同様.
いづれも定理4.11の系および定理3.7の系による.さらに,
に一致するので,恒等写像が所期の同相を誘導する:
と定める.このとき,任意の
が成り立つ.実際,命題18より
であり,
が成り立つ.
命題22により命題14の別証明が得られる:
基点つき空間
が成り立つ.
を得る.
命題22は次の形に一般化できる:
が成り立つ.
を商写像とする.仮定より
を一点につぶす同値関係に一致するので,
が成り立つ.
命題23の証明よりつぎがわかる:任意の
を誘導する.
が成り立つ.
のいづれかが成り立つならば,同相
が成り立つ.
全射連続写像
を考える.
に一致するので,恒等写像が所期の同相を誘導する:
が成り立つ.
命題24と縮積の可換性より
が成り立つ.
非負整数
が成り立つ.実際,命題18,例7と
が成り立つ.或いは例9と命題23より
を得る.
参考文献
他の人のコメント
現在のページ
商空間の例- 錐
- 懸垂
- 結
- 結の結合性について
- 等化結と誘導結
- 誘導結の結合性
- 等化結の結合性:コンパクトハウスドルフ空間の場合
- 等化結の結合性:局所コンパクトハウスドルフ空間の場合(未)
- 基点つき空間
- 被約錐・被約懸垂
- 楔和
- 縮積
- 参考文献
